That wasn't so tough. The biggest problem was, what size do I make the outer circle and the inner circle? So, I recalled my many essays on "Robert Gordon's 7 Days" ("The Tunnel Thru the Air"). RG circumvents the earth which was a 33K mile trip. When you parse that trip , you find he traveled about 18K miles east/west and 18k miles north south. Interesting, 18K miles is the circumference of the earth at about the same parallel as New York City. No, Mr. Noxious Poster, the earth is not 24,901 miles in circumference everywhere. In fact, the earth is not a sphere but a spheroid with slightly flat poles. But I diverge.
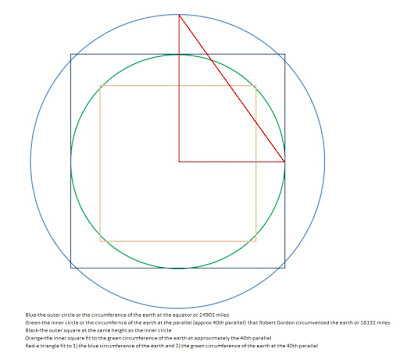
What if we considered the outer triangle to be the circumference of the earth at the equator or 25K as viewed from the north pole. That's the blue circle. And the inner green circle is RG's east/west mileage or 18K.
Now draw the outer black square exactly within the outer circle. My gosh, it is almost perfectly encloses the green inner square. There is something very familiar about the outer circle, outer square and inner circle. Where did I see those 3 figures?
Oh well, let's add the inner triangle. Mr. Gann was a Mason so we need a 90* triangle. And, well, two concentric circles. Aha, squaring the circle (1)!!!! Configure the triangle to vertically connect the center of both circles to the outer circle and horizontally connect the center to the inner circle.
[Not so fast Tanto; there is one and only one triangle that squares the circle. It is the Kepler triangle which is a geometric progression with sides of 1, sqrt(Phi) and Phi. A Pythagorean is but a lowly right triangle with an arithmetic progression of 3, 4 and 5.]
Now do the math. The radius of the larger circle/earth at the equator is 3959 and of the RG circumference is 2885. The hypotenuse would be 4808:
Put those 3 numbers, which constitutes the red triangle in the first illustration above, on an Excel spreadsheet and reduce them. I'll cut to the chase, at least, the first chase. 2886 divided by 961 is 3.0, 3959 divided by 961 is 4.1 and 4899 divided by 961 is 5.1.
The triangular relation of earth's equatorial circumference to its circumference at about the 40th parallel is the Pythagorean 3-4-5 triangle.
It would be hard for me to believe Mr. Gann's riddle of the triangle, circles and squares is not intrinsically related to the riddle of "Robert Gordon's 7 Days." But that's me. The noxious poster would say "I read all of Robert Gordon's 7 Days and it is meaningless. It doesn't have any dollar signs anywhere in it. So you can't make a prediction." He's so cute.
Now I remember. The first illustration above looks uncannily like that presented by Bradley Cowan on page 45 of "Four Dimensional Stock Market Cycles and Structures." In it he explains the geometry of the circle and the square defining the doubling of the square, among other things. Really? Page 45 or half the 90* angle of the right triangle and Mr. Gann's hallmark of dividing time. Again I diverge.
So what's Robert Gordon's relation to the fourth dimension and markets as teased? There aren't any dollar signs. Such a powerful argument.
Does the first illustration look like some of the representations of Mr. Gann's legendary "Square of 9?" Yes it does. You have the inner square which would be populated by prices (a proxy for space). You have the inner and outer circle representing time and on which people will mystically place months or days or trading hours. And you have a triangle which people, supposedly with insight, configure as an isosceles or as a right triangle that touches the same outer circle but not the inner circle and the outer circle. Its all random, "I think it should be this way or that way but I don't have a scientific reason."
All that noted, I propose RG's relation to the fourth dimension and markets is this. The dimensions of RG's trip imposed on the "Square of 9" shows a relation between time at the 40th parallel - where markets trade in NYC - and the equator - where terrestrial time is defined. And the circular nature of time on the "Square of 9" is related to the squareness of space, as represented by market price by the triangle. The triangle, and more pointedly, the Pythagorean triangle defines the inextricable relation of time and space. After all, we know in physics that space and time are so related. We find confirmation of that notion in the current issue of Scientific American which is dedicated to 100 years of Einstein's general relativity. I'd previously read this quote but had to go back and re read several of the articles to find it:
Did I find it on pager 45 as I did the Cowan illustration? No, I found it on page 44…but…page 44 has an indented illustration of "Relativity Primer" which is split with page 45. Page 45 is a subset of page 44 and not an independent page. Just my X-files instinct showing itself.
This is where it gets really interesting. Einstein proved to himself that his equations and theory was correct when in 1915 he applied them to the astrophysics problem of Mercury's perihelion drift. In 100 years, Newton's laws would miss predicting the orbit of Mercury by 43 seconds. 43 seconds in 100 years, yet, an error is an error. Einstein found his formulaic representation of general relativity was perfect.
Similarly, I believe that Mr. Gann had proven to himself that the markets were proof of the physics of the fourth dimension. And the correct application of those physics may have been the 'Square of 9.' Of course, I do not know how to correctly use the 'Square of 9' but I know others do not. As Socrates argued in 'The Apology' something along the lines of "I know I am not as wise as the Gods, but I am wiser than other men who believe they are wise. I am wiser because I know what I don't know. They don't." After studying dozens of texts for untold hours, I cannot find any that prove they know the scientific method that might be at work in the 'Square of 9.'
I call this essay part 1 because it is the terrestrial or special relativity application of the 'Square of 9,' It is named thus because it involves the two circles of the equator and Robert Gordon's circle at about the 40th parallel. It relates to the shorter time periods and prediction based on the earth's rotation alone. It involves special relativity because special relativity is concerned with the geometry of spacetime in the absence of differing gravitation or acceleration; the Minkowski space. All of us on the surface of the earth are subject to pretty much the same gravity. Absent our being on a jet or falling from a building, we're not subject to acceleration. The only relative difference between us is our location. Our relative locations do create a difference in special relativity. A person sitting on a park bench at Battery Park is traveling at a speed of approximately 785 miles per hour (the circumference of the earth at the latitude of NYC divided by 24 or 18832 / 24) which a person on the exact equator is traveling at 1037 miles per hour (the circumference at the equator divided by 24 or 24910 / 24).
This part 1 is 1 of 3 because there are two other fundamental applications of the Square of 9 in my estimation. The second would be the general relativity model caused by the moon's gravitation. Since the moon presents the second largest gravitation effect on the surface of earth (by virtue of its proximity despite its diminutive size), it creates the second greatest gravity well. The outer circle would be the moon's cycle and the inner circle would be the earth's rotation….that's my guess.
And part 3 of 3 would be 'Square of 9' model based on the general relativity model of the sun's gravitational effects on the earth. The outer circle would be the sun's period and the inner circle would, again, be the earth's axis. But again, that's a guess at this point.
What is evident to me is, these two distant points in Mr. Gann's writings (that the fourth dimension is proven by market movements and Robert Gordon's 7 days) may well teach the reasoned and scientific working of the 'Square of 9.' Not the mystical "He brought it back from the far east." It also suggests to me, Mr. Gann was fully aware of the nature of both Special Relativity and General Relativity. Not only that, he applied Special Relativity to the stock markets as early as the 1909 Ticker Interview. Remember the stories of his trading…that he had with him only a 'Square of 9 chart' and his ephemeris? He not only knew and understood Relativity, but could apply it to the market…..before Einstein.
I wish I could really cut to the chase and know what the 'Square of 9' meant and how to use it. I don't. But with each new consideration of merit perhaps I get that much closer to knowing. It's a process to use a tired colloquial BS phrase. But its a method not unknown to those chasing an answer:
"Data, data, data. I can't make bricks without clay."
Jim Ross

No comments:
Post a Comment
Note: Only a member of this blog may post a comment.